Univ. of Minnesota
Abstract. Boyle's law is the epitome of lawlike science in the classroom. Yet Boyle's law is not universal and invariant, as implied by the term 'law'. It does not hold at high pressures, low temperatures, or low volumes. It is based on constant temperature. It varies for different gases. Boyle's law—and other scientific "laws"—are not lawlike at all. An alternative is to teach a more complex view of nature, exhibiting only patches of regularity. In following scientists, teachers may focus on experiments as concrete models, such as Boyle's J-tube. Science education might well be ideally rooted in concrete apparatus and teaching experimental and analogical reasoning, not laws. Introduction Presented with a title like "Teaching Science Lawlessly," you may be envisioning science red in tooth and claw, chaotic nature careening out of control, or gangs of science teachers roaming through school hallways wieldng huge Leyden jars ready to unleash electrical havoc on unruly students. That's not a bad image to start. Let me couple that image with an appreciation: namely, a recognition of the achievements of the community in Oldenburg, Germany, under the Falk Reiß's vision and leadership. I hope to elucidate how an image of lawlessness might serve this end: ultimately, as a tribute to the pioneering work there. Towards that end, my central focus, and the occasion for analysis and commentary, is one of the most standard of benchmark concepts in introductory chemistry or physical science: Boyle's law. I focus on this law primarily because it is hard to find a science curriculum without it having a central place. It is central, I think, not because it informs students about how gases behave. Rather, it is a cultural icon that epitomizes the fundamental concept of a law. My aim is to profile an alternative that may better reflect science in real practice. Most of you are no doubt familiar with the historical context of Boyle and his law. Boyle was interested in the "spring of air." Air exerts pressure when compressed. Borrowing from a device of Otto Guericke, Boyle enlisted Robert Hooke, to build an "air pump." They did not generated a true vacuum, but the vessel did exhibit extremely low pressure. In 1660 Boyle published his findings on its many effects — on magnets, sound transmission, sealed bladders, burning candles, the life of small animals, and more. In response to criticism, Boyle further demonstrated the strength of the spring of air. He and Hooke compressed a small amount of air trapped in the end of a glass J-tube with increasing amounts of mercury. In a similar second set of experiments, they used the new air-pump to draw up the column of mercury to dilate the trapped air. In both cases, they recorded the volume of the trapped air and the corresponding height of the column of mercury (an indirect measure of its weight, or pressure). Students even today can graph his figures and see the inverse relationship of pressure and volume (Conant 1957). There are many ways to express Boyle's law. My analysis will be based on a narrow, but explicit version:
The change in pressure is inversely proportional to the change in volume. (As the volume increases, the pressure decreases, and vice versa.) What's Not in Boyle's Law The concept of scientific laws, such as Boyle's law, has a venerable tradition. Laws are empirically substantiated generalizations or regularities. For many, they are the basic units of scientific knowledge. Laws reflect a conception of nature as law-like or machine-like, as expressed in the mechanical philosophy, famously advocated by Boyle (Sargent 1995). Nature may thus be described by reducing phenomema to their parts and the laws that govern their interaction. Elucidating these laws is widely portrayed as the major goal of science. Familiar examples of laws might also include Snel's law of refraction, Galileo's law of the pendulum, Newton's laws of motion, Ohm's law of electrical resistance, and Mendel's law of independent assortment. As typically presented and interpreted, scientific laws are invariant and universal (Hempel 1966, 54, 58; Ziman 1978, 32; Kosso 1992, 52-60, 190; Woodward 2003, pp. 167, 236-238, 265-266). Indeed, their very universality and invariance accounts for their value and authority as generalizations. Laws accordingly seem to earn central place in science education. As Boyle's data show, Boyle's law certainly holds true in Boyle's J-tube. But is Boyle's law universal? Is it invariant? Is it truly lawlike? Does it hold in all cases? The answer is "no." It depends on context. At high pressures, the direct inverse relationship of pressure and volume breaks down. Henri Regnault (in 1852) and later Louis Cailletet noted this variation two centuries after Boyle's work. In modern terms, we might say that there is a limit to compression. Under very high pressures, a gas begins to behave more like a liquid than a gas. The scope of Boyle's law is limited. It only holds in the restricted domain of pressures up to approximately ten atmospheres. Never mind that these pressures are infrequently encountered in daily human life: the law is plainly not universal. Perhaps the behavior of gases at high pressures is a rare, minor exception. Suppose one stipulates explicitly, then, that only certain pressures apply:
For all pressures not substantially greater than zero, Is Boyle's law universal now? Well, no. At low temperatures, the the inverse relationship breaks down, as well. Historically, this was noted by Thomas Andrews in the 1860s (Andrews 1869).
Here, another context qualifies scope. But this case also introduces something unexpected: a new variable. The equation or formula does not even refer to temperature. An experimenter, for example, would need to be aware of temperature, if only to ensure that the temperature was not extremely low. The simple expression of Boyle's law hides this relevant variable entirely. Well, then, let us add both these conditions, or provisos:
For all temperatures well above zero, Is Boyle's law "fixed" now? For example, with the provisos of scope, is the law now invariant? Well, no. Temperature is indeed important. —Not just the range of the temperature, but also its holding constant. As Boyle himself (and others of his era) noted, gas pressure is sensitive to changes in temperature, as exemplified in several apparatus for measuring temperature. A few decades later, of course, by Jacques Charles (in 1787) and Joseph-Louis Gay Lussac (1802) formalized this in yet another law, which now bears (alternately) their names. Hence, while one may address the relationship of pressure and volume independently of temperature, temperature is nonetheless relevant. Constant temperature is a boundary condition. Sometimes it is expressed as a ceteris paribus clause: "all else being equal." But not all other things need to be equal. The amount of illumination, the relative motion of the system, or gravity exerted on it, have no effect on gas behavior, so far as we know. No one need stipultate these as boundary conditions or imagine them in a ceteris paribus assumption. So specifying what must remain constant is important indeed if one expects the law to hold. The invariance of Boyle's law, ironically, depends on context. Well, let us now add our additional boundary condition:
For all temperatures well above zero,
Is Boyle's law expressed fully now? No, still not yet(!). If the volume is very low (or the density very high), the volume of the gas molecules relative to the volume of the space between them becomes significant. Intermolecular interactions (London forces) become relevant. The behavior of the gas changes noticeably. Moreover, because the size of the gas molecule matters, the variation is specific for each gas. Yet another factor qualifies Boyle's law. Even the qualifications are not regular. Moreover, any gas with strong polarities may also exhibit intermolecular forces, although of another sort.
To be accurate, or realistic, Boyle's law must sacrifice universality. Boyle's law, like so many others, is only "lawlike" when qualified:
∀ To0 & ∀ P ~o0 & ∀ Vo0 & ΔT=0 ⇒ ΔP ∝ 1/ΔV
For all elastically compressible gases, A critical analysis of Boyle's law exposes and highlights this double irony: universality comes at the cost of limited scope; invariance, only with conditions. The lawlike world of "for all..." is inseparably coupled with a set of contingent "if-and-only-if"s. This is the lesson typically missing when teaching Boyle's law: what's not in Boyle's law — a world full of context and contingency, not simple rules. Another irony lurks in Boyle's law. That is, Boyle himself never stated it. He certainly produced data commensurate with the law, yet he did not write an equation for the relationship. He surely understood the mathematics of "what the pressure should be according to the hypothesis" (Boyle 1662), yet he was generally disinclined to characterize nature mathematically (Shapin 1996, pp. 333-338). Boyle also embraced the concept of laws in science: he advocated mechanical philosophy as well as the theological framework of construing nature in terms of inherently divine laws (Boyle 1661). Yet Boyle did not regard his findings about the condensation and rarefaction of air as a universal law in the sense now accepted. Rather, he referred to the spring of air more modestly, as a 'habit of nature' or 'custom of nature': local, perhaps contingent, in nature (Boyle 1661; Sargent 1995; Shapin 1996, pp. 328-330, 338-350). He was well aware, for example, that temperature and "atmospheric tides" affected the Torricelli tube, today's mercury barometer (Boyle 1660, pp. 65, 123, 133; 1682, p. 50). Boyle's law is not strictly Boyle's. Teachers might thus profitably reflect on Boyle's own modest posture. Rampant Lawlessness One may well be tempted to imagine that there is no need for such caution, that Boyle's law is an exception and that most scientific laws are universal and invariant as promised. Not so. Consider Galileo's "law" of the period of the pendulum:
t = 2π√(l/g)
(where x is the angle of swing). This may be universal, but it is rarely used. It cannot be solved analytically and requires iterative substitution even to approximate solutions. But even this expression assumes that the mass is concentrated on a single point and that no friction affects either the fulcrum or the interaction of the pendulum and its medium. These are not just boundary conditions, but unrealizable idealizations, as all the physicists here well know. Ohm's Law of electrical resistance, too, has numerous exceptions: for example, at high current densities. Many common materials "violate" Ohm's Law: temperature-sensitive resistors (such as filaments in incandescent light bulbs, or sensors in digital thermostats); air (whose threshold resistance results in bolts of lightning); diodes (common electronics components), light-sensitive resistors, piezoelectrics (used in touch-sensitive switches), weak electrolyte solutions, varistors and high-vacuum electron tubes, as well as other more technical variants. Newton's Laws of Motion do not apply at relativistic velocities (approaching the speed of light), and (like the pendulum law) exclude friction. Mendel's Law of Independent Assortment breaks down, as most students learn, for genes linked on the same chromosome. Snel's Law of Refraction does not apply for 'Icelandic spar', or calcite. In all these cases, laws seem very unlawlike. Scope circumscribes universality. Boundary conditions and exceptions limit invariance. That is, context matters. One need not contend that these or other scientific laws are completely invalid. Nor need one discount their informativeness. The emphasis, instead, is on context. Few professional scientists or philosophy of science—nor likely anyone here—deny that laws are ultimately contextual. For example, Toulmin (1960, pp. 31, 63, 78-79, 87) underscores that laws have particular scope of application, or domains. He, along with Kuhn (1970), suggest that articulating this scope is a major function of scientific research. Still, Toulmin and others contend, the provisos are not part of the law. Of course, that is a philosophical sleight of hand. Only by erasing these conditions does a law acquire the illusion of universality. Laws, if not false, are illusory. They attain law-like status only by arbitrary specification of background conditions—a control which may be locally informative, but not universally valid. Context depends on perspective. Interpretations of foreground and background rely on convention. So one may ask: why are laws central, and the exceptions "exceptions"? Why regard "laws" as primary? I am inviting a gestalt switch whereby cases now framed as "outside the scope" or "not within the boundary conditions" are framed instead as inside the system.
One denies laws privilege. Accordingly, the unlawlike earns parity. Boyle's law becomes a special case: no less true, but narrowly so, contingent on a particular context. It demarcates a patch of local regularity. The alternative perspective embraces the whole, of which Boyle's law is only a part. An unrepentantly staunch realist does not present an idealized gas law as even a simplification of all real cases. Laws are the exception, not the rule. They require special explanation, just as they need special experimental conditions to be observed in a lab. Laws are local, not universal. Generalities are contingent, not invariant. Accordingly, one may be impressed by an apparatus where Boyle's law is observable, such as Boyle's J-tube. A change in volume is observed only when the system is closed. In an open system, such as the atmosphere, a gas under pressure does not change volume. It moves: hence, wind! Indeed, the cases where Boyle's law holds are few: pistons (say, in internal combustion engines), industrial boilers, some ventilation systems. Boyle's law is not "basic" at all. It is an esoteric scientific fact. Few students will ever need to know or apply Boyle's law in either their personal or professional lives. Yet it is de rigeur for science education. The ultimate lesson of Boyle's law is not about how gases behave: it is about laws and characterizing nature as essentially lawlike — and that lesson is grossly misleading. We live in a world where weather happens. We need to teach equally about non-lawlike, or lawless, nature. Lawless Causality To accommodate lawless science, one needs to readjust the basic framework of causal thinking. But how can one think causally without a law? (Is it even possible?!) The mere image of lawless science, of course, bristles with potentially disruptive images of anarchy. It reeks of chaos and disorder. But the effect is purely linguistic. Consider the contradiction, for example, in claiming that a natural phenomenon violates or deviates from a law of nature. The language links law and order in the civil realm. We need to ensure that our thinking about science is not biased by meanings in cultural contexts. A lawless nature may seem to imply indeterminism. However, science without laws need not be any less deterministic. Lawless science fits with an alternative conception of causality. Conventionally, laws reduce the world into component parts and causes. Laws are the basic units. Laws are like billiard balls. Each moving billiard ball expresses a mechanical vector: a cause. One ball hits another with an observable effect. The second ball may, in turn, hit another, and then perhaps another, leading to a causal cascade. Laws lead to thinking in terms of causal chains. Even where a cue ball hits a whole rack of fifteen balls, with scattering effects, all calculable (in principle) from the cue ball's original vector. The complex event is explained by compounding individual causal events, layering one cause upon another. This reductionistic view is nicely exemplified, of course, by the kinetic theory of gases, the modern explanation for Boyle's law. Gas molecules act like billiard balls colliding in three-dimensional space. The pressure is a collective effect of all the movements of the individual gas molecules, each with their own discrete contributing cause. The alternative conception of causality is more holistic. It underscores multiple simultaneous causal factors. It resonates with a state system view of causality. That is, effects are not attributed to individual causes, or even to a composite of overlapping causes. Rather, effects are due to the state of the entire system. One identifies the whole as "the" cause, differentiating—but not privileging—individual elements. Pressure does not increase merely due to a decrease in volume, as suggested by Boyle's law. Rather, pressure increases due to the change in volume and extant pressure and temperature and overall volume and type of gas — even when the temperature does not change. The behavior of gases is always, not just sometimes, multivariate. Just so for pendulums, electrical resistance, inheritance, etc. For further perspective on framing causality, consider the playful devices of Rube Goldberg. He delighted viewers by imagining how a modest action, such as emptying an ice cube tray or cooling hot soup, resulted from an elaborate and improbable series of events. The humor arises chiefly from the convoluted causal pathway, underscoring the (otherwise humorless) association of simple causes with simple effects. Goldberg's elaborate mechanisms also highlight causal lineages, often labeled in an alphabetical sequence. At the same time, his devices are funny precisely by being incredibly contingent. If a rolling ball is not preset, a falling hammer not lifted, a spring not prewound, the causal cascade is interrupted. The design elicits a fun anticipation of several successive "only-if"s. The causal scenario is exceptionally fragile. Action depends on the whole structure at once. The arrangement and set-up—the context—is critical. The causal lineage is merely an artifact of the causal structure—primed throughout, but not triggered. Goldberg's baroque and apparently silly devices, then, offer sophisticated commentary on reductionistic causal perspectives. Their whimsy helps celebrate a contingent view of causality. Consider again the reductionist's billiard balls. Here, the status of the whole billiard table is important. One cannot assume that balls are intially at rest: the system may already be complex and active, not passive in response. Nor can one assume the balls to be of the same material (like different gases perhaps?). They need not react in uniform ways: identical vectors underdetermine a collision outcome. The billiard table environment also matters: it may not be level, or remain level (anymore than the temperature of a gas). A tilted table affects all the components at once, even when the balls are not colliding. Some causal factors may undulate the surface, generating different local conditions across the table, leading again to different responses for apparently similar collisions. Some balls may contain iron, so changes in the magnetic field (context) may matter more than the interactions of individual balls (parts). With uniform balls on a level billiard table—which stays level—simple interactions may indeed be discernible. But that is a special case. The state system view looks well beyond the billiard balls—or individual gas molecules—as component causes. It emphasizes the whole and the contexts of the parts. Let me note, at this juncture, that this presentation is derived and adapted from a larger project of mine, still in progress, on "The Gender of Boyle's Law." One could probe the history of laws in science and show that the approach is based on theology and a world view that values technology, control and mechanical philosophy (Steinle 2002). Or one could delve into the growing literature on models and mechanisms (Glennan 1996; Machamer, Darden and Craver 2000; Bechtel 2006), or on lawless nature (Cartwright 1999). Today, however, I want to profile further the concrete alternatives of lawless causality. From Laws to Material Models So: strip the lawlike status from Boyle's law and it may seem as if nothing remains. What remains, of course, is Boyle's J-tube as an exemplar and concrete model, Kuhn's (1970) core sense of paradigm (see also Giere 1995, 1999). A focus on models and particularity underscores the importance of material culture and experimental systems in investigation, as profiled recently by Hans-Jorg Rheinberger (1996). Here, one does not appeal to general laws. Rather, one compares other cases to Boyle's original. To the extent that the conditions are the same — including pressure, temperature, etc. — one expects to find similar behavior. The reasoning is primarily analogical, not deductive. It is also direct, not indirect. One does not detour through a general law, first by abstracting Boyle's J-tube, then by deciding whether a new case is an authentic example of the general law. Reasoning from case to case does require, however, clear attention to the variables and details of context. One quickly learns the basis and limits of similarity, an integral part of the exemplar, or paradigm. The lawless alternative to Boyle's law is Boyle's J-tube. It is eminently teachable. Although lawless science challenges lawlike thinking, the legal system (on a cultural level) provides an instructive metaphor. In practicing law, especially in juridicial contexts, one distinguishes between statute (or code) law and common law. Statute law is based on rules, or codes: like the laws of nature. One assesses actions in reference to the law's general and explicit statements. One reasons deductively. Common law, by contrast, is case-based. One assesses actions based on precedent, or similar cases encountered in the past. Interpretation emphasizes the basis for similarity. One reasons chiefly analogically. Of course, numerous variables, or bases for similarity, are usually possible. The effectiveness of an analogy may be highly contingent. Context plays a major role. Yet the multitude of benchmarks can be beneficial, especially in interpreting complex cases. Under statute law, statutes may overlap and indicate conflicting interpretations. Case-based reasoning can often resolve this. Both frameworks provide viable systems of law and interpreting justice. Imagine lawlike science resonating with statute law, "lawless" science with common law. Accordingly, Boyle's J-tube is significant primarily as a precedent. To apply Boyle's law, for example, one depends on being able to match the particular conditions of Boyle's J-tube. It is no surprise, perhaps, that scientists who subsequently revised Boyle's law typically followed Boyle's model by using mercury in J-tubes. For example, with the construction of the Eiffel Tower, Louis-Paul Cailletet was able to build and support a column of mercury of unprecedented height. With it, he could examine the same system, but now with pressures up to a hundred atmospheres. He studied the limits to Boyle's law by probing variations in the original set-up. Indeed, scientists often focus primarily on experimental systems, rather than on specific concepts (Rheinburger 1996). The lineage of investigation is often shaped by the materials and opportunities at hand, not by a theoretical master-plan. While Boyle's J-tube may be local and contextual, it is no less valuable on that account. Indeed, its particularity functions to guide reasoning through analogy—and to help keep it in check. Historically, Boyle's J-tube —not the law— may be the more fundamental benchmark, and that may serve as the critical clue for what one teaches about the nature of science. How, indeed, does one adapt teaching to accommodate the wider view of causality, to open student awareness of the context of laws and of lawless nature? The ultimate import of my comments, here and at this time (under these particular circumstances), may finally be clear. If one were to assemble a science education curriculum founded on the importance of material systems and experimental exemplars, one could hardly do better than follow the model set by this institution. Here is a paradigmatic environment for teaching science without laws. In teaching with historical perspectives and replicas of original apparatus, the role of precedent in science is made clear. Students equally learn the role of concrete experimental models. While there are other aims at work here, the instructional framework supports appreciation of scientific reasoning and practice beyond laws. Let me highlight (perhaps celebrate) just two examples of what one may learn from this approach. Each underscores the importance of understanding the materialty, not just the concepts of experimental practice. Recreating the work of Joule on the mechanical equivalent of heat would seem simple enough: after all, it's been done before. However, efforts here led to important discoveries. One factor they traced was particular dimsneions of the paddle that rotated through the water chamber of the calorimeter, an apparently modest variable of unsuspected significance (Heering 1992). Joule's findings were context-dependent indeed. In other work, they tried to replicate Coulomb's work on electrostatic attraction. Work with the apparatus was so delicate that they ultimately questioned whether Coulomb could have produced the results he reported (Heering 1991). Both cases echo the lesson that the materiality of experiment is more complex than the simple equations and laws that educators often present as the foundation of science. One need not assume that the Oldenburg group aims explicitly or even intentionally to foster understanding of lawless science. Indeed, as these two examples illustrate, a major goal seems to be modeling how the classic laws developed historically. The laws are still prominent landmarks of science. But this is not inconsistent with Boyle's modest posture, or an appreciation of the limited context of laws. I want to highlight how the Oldenburg model implicitly guides students through experimental thinking and thus promotes the type of understanding I am advocating. I am hoping that others may learn from the experience here and, with instruments in hand, fruitfully pursue the prospects of teaching science lawlessly. References
| ||||||||||||||||||||||||||||||||||||
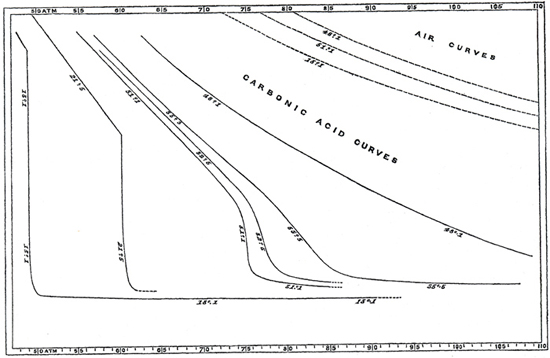
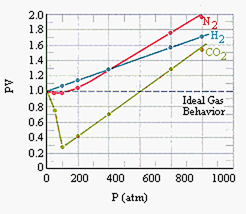Such gases—carbon dioxide, for one—also vary from the simplified "ideal." Ultimately, then, we must also take into account the nature or identity of the gas (or gases). Johannes van der Waals investigated these various dimensions of gas behavior in the 1870s and 80s, as well as the nature of some of the inter-molecular forces, work that was recognized by a Nobel Prize in 1910. One can "correct" for the subtleties of molecular size and interactions, but the corrections (known as van der Waals constants) differ for each gas. Thus, even his now well known generalized form of the gas law included variables specific for each gas:
